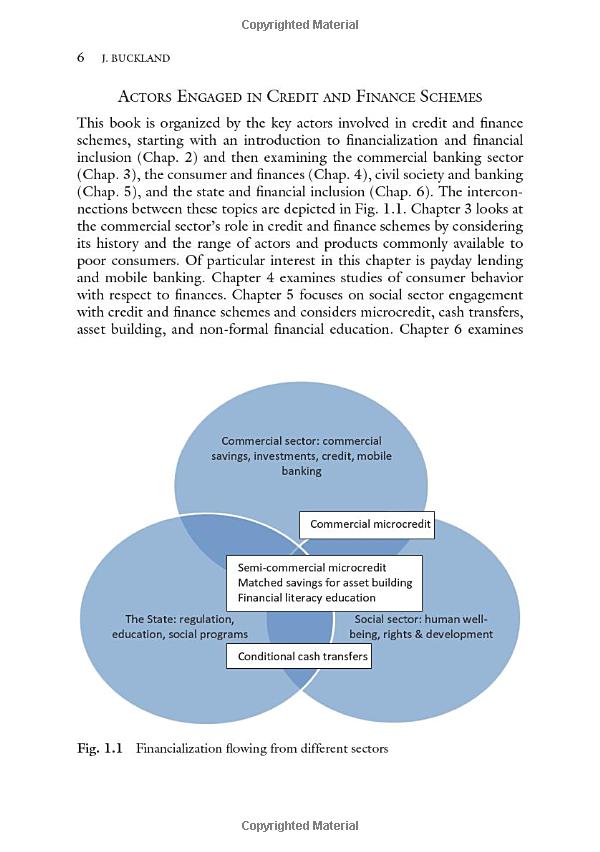
Mastering Your Finances: A Comprehensive Guide to the Loan Calculation Formula

#### Understanding the Loan Calculation FormulaThe **loan calculation formula** is a crucial tool for anyone looking to borrow money, whether for a home, a……

#### Understanding the Loan Calculation Formula

The **loan calculation formula** is a crucial tool for anyone looking to borrow money, whether for a home, a car, or personal expenses. This formula helps borrowers understand how much they will need to repay each month, the total interest paid over the life of the loan, and the overall cost of borrowing. By mastering this formula, you can make informed financial decisions and avoid falling into debt traps.

The basic loan calculation formula is often represented as:

\[ M = P \frac{r(1 + r)^n}{(1 + r)^n - 1} \]

Where:

- \( M \) = total monthly payment

- \( P \) = principal loan amount

- \( r \) = monthly interest rate (annual interest rate divided by 12)

- \( n \) = number of payments (loan term in months)

#### Breaking Down the Components

To effectively use the **loan calculation formula**, it’s essential to understand each component:

1. **Principal Loan Amount (P)**: This is the initial amount of money borrowed. For example, if you take out a mortgage for $200,000, this amount is your principal.

2. **Monthly Interest Rate (r)**: This is the annual interest rate divided by 12. If your loan has an annual interest rate of 6%, your monthly interest rate would be 0.5% or 0.005 in decimal form.

3. **Number of Payments (n)**: This refers to the total number of monthly payments you will make. For a 30-year mortgage, \( n \) would be 360 (30 years x 12 months).

#### Calculating Your Monthly Payments

Let’s say you are considering a loan of $250,000 with an annual interest rate of 5% over 30 years. First, convert the annual interest rate to a monthly rate:

- Annual Interest Rate = 5%

- Monthly Interest Rate (r) = 5% / 12 = 0.4167% = 0.004167

Next, calculate the number of payments (n):

- Loan Term = 30 years

- Number of Payments (n) = 30 x 12 = 360

Now, plug these values into the loan calculation formula:

\[ M = 250000 \frac{0.004167(1 + 0.004167)^{360}}{(1 + 0.004167)^{360} - 1} \]

Calculating this will give you your monthly payment. In this example, the monthly payment would be approximately $1,342.05.

#### Understanding Total Payments and Interest

Once you have your monthly payment, you can easily calculate the total amount paid over the life of the loan and the total interest paid.

- **Total Payments**: Multiply your monthly payment by the number of payments:

Total Payments = Monthly Payment (M) x Number of Payments (n)

- **Total Interest Paid**: Subtract the principal from the total payments:

Total Interest = Total Payments - Principal Loan Amount (P)

Using our previous example, the total payments over 30 years would be:

Total Payments = $1,342.05 x 360 = $482,138

Thus, the total interest paid would be:

Total Interest = $482,138 - $250,000 = $232,138

#### Benefits of Using the Loan Calculation Formula

Using the **loan calculation formula** provides several benefits:

1. **Budgeting**: Knowing your monthly payment helps you budget your finances better.

2. **Comparison**: You can compare different loan offers by adjusting the principal, interest rate, and loan term.

3. **Financial Planning**: Understanding the total cost of borrowing allows for better financial planning and saving strategies.

#### Conclusion

The **loan calculation formula** is an invaluable tool for anyone considering taking out a loan. By understanding how to calculate your monthly payments, total payments, and interest, you can make informed decisions that align with your financial goals. Whether you are a first-time borrower or looking to refinance, mastering this formula will empower you to take control of your financial future.